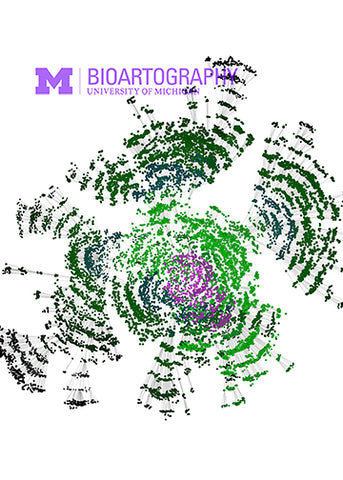
Edward Flach, Graduate Student, Mathematics, University of Michigan

In 1952, Alan Turing proposed that the development of patterns in organisms could be modeled by a mathematical equation. Examples are simple to find: spots on leopards, stripes on fish and shells, even the coloring of pandas! With the possibility that mathematics could help to explain biology, there has been much investigation into this. We have been exploring the model in an abstract way, perturbing Turing’s theory to examine its robustness. Here the horizontal axis is space; the left might be the head of an organism and the right the tail. The vertical axis is time, increasing from bottom to top. The morphogen expression is triggered at the beginning and an oscillatory pattern develops along the entire length of the animal. At high concentration of the morphogen, cells would fix a pigment.